Question

30.0 mL of 0.20 M AgNO, are added to 100.0 mL of 0.10 M HCI in a thermally nsulated vessel. The following reaction takes place: Ag (aq)+ Cl (aq)AgCI (s) The two solutions were initially at 22.00°C and the final temperature was 22.80 C Calculate the heat of this reaction in k.Jimol of AgCI formed. Assume a combined mass of 120 g and a specific heat capacity of 4.18 JK-'g for the reaction mixture.

Answer 1

Answer : The heat of this reaction of AgCI formed will be, 66.88 KJ

Explanation :

First we have to calculate the heat of the reaction.

`q=m* c* (T_(final)-T_(initial))`

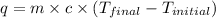
where,

q = amount of heat = ?

`c` = specific heat capacity =
`4.18J/g.K`

m = mass of substance = 120 g

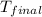`T_(final)` = final temperature =
`22^oC=273+22=295K`

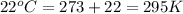
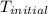`T_(initial)` = initial temperature =
`22.8^oC=273+22.8=295.8K`

Now put all the given values in the above formula, we get:

`q=120g* 4.18J/g.K* (295.8-295)K`

`q=401.28J`

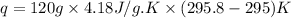
Now we have to calculate the number of moles of
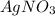`AgNO_3` and
`HCl`.

`\text{Moles of }AgNO_3=\text{Molarity of }AgNO_3* \text{Volume of solution}`

`\text{Moles of }AgNO_3=0.20mole/L* 0.03L=0.006mole`

`\text{Moles of }HCl=\text{Molarity of }HCl* \text{Volume of solution}`

`\text{Moles of }HCl=0.10mole/L* 0.1L=0.01mole`

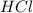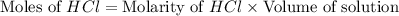
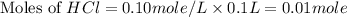
Now we have to calculate the limiting reactant.

The balanced chemical reaction will be,

`AgNO_3+HCl\rightarrow AgCl+HNO_3`

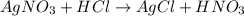
As, 1 mole of
`AgNO_3` react with 1 mole of HCl

So, 0.006 mole of
`AgNO_3` react with 0.006 mole of HCl

From this we conclude that,
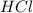`HCl` is an excess reagent because the given moles are greater than the required moles and
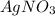`AgNO_3` is a limiting reagent and it limits the formation of product.

Now we have to calculate the moles of AgCl.

The given balanced reaction is,

`Ag^++Cl^-\rightarrow AgCl`

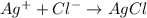
From this we conclude that,

1 mole of
`Ag^+` react with 1 mole
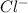`Cl^-` to produce 1 mole of
`AgCl`

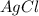
0.006 mole of
`Ag^+` react with 0.006 mole
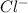`Cl^-` to produce 0.006 mole of
`AgCl`

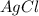
Now we have to calculate the heat of this reaction of AgCI formed.

As, 0.006 mole of AgCl produced the heat = 401.28 J

So, 1 mole of AgCl produced the heat =
`(401.28)/(0.006)=66880J=66.88KJ`

Therefore, the heat of this reaction of AgCI formed will be, 66.88 KJ