Question

(1.)Find the slope of the line that passes through the given pair of points. (If an answer is undefined, enter UNDEFINED.) (?a + 3, b ? 3) and (a + 3, ?b) *******(2.)If the line passing through the points (a, 1) and (6, 5) is parallel to the line passing through the points (2, 7) and (a + 2, 1), what is the value of a?

Answer 1

1. The slope of the line is
`m=(-2b+3)/(2a)`.

2. The value of a is 18.

Explanation:

If a line passes through two points, then the slope of the line is

`m=(y_2-y_1)/(x_2-x_1)`

(1)

It is given that the line passes through the points (-a + 3, b - 3) and (a + 3, -b). So, the slope of the line is

`m=(-b-(b-3))/(a+3-(-a+3))`

`m=(-b-b+3)/(a+3+a-3))`

`m=(-2b+3)/(2a)`

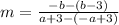
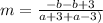
The slope of the line is
`m=(-2b+3)/(2a)`.

(2)

If the line passing through the points (a, 1) and (6, 5), then the slope of the line is

`m_1=(5-1)/(6-a)=(4)/(6-a)`

If the line passing through the points (2, 7) and (a + 2, 1), then the slope of the line is

`m_2=(1-7)/(a+2-2)=(-6)/(a)`

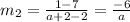
The slopes of two parallel lines are same.

`m_1=m_2`

`(4)/(6-a)=(-6)/(a)`

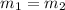
On cross multiplication we get

`4a=-6(6-a)`

`4a=-36+6a`

`4a-6a=-36`

`-2a=-36`

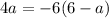
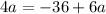
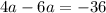
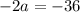
Divide both sides by -2.

`a=18`

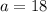
Therefore the value of a is 18.