Answer:
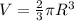
Explanation:
The Volume of a cone is by definition 1/3 of the volume of a Cylinder. In this question, the height equals to diameter (2R).
So, We have:
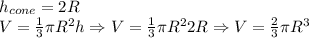
We conclude that under this circumstance, a cone with a height equal to its diameter will turn its volume to be equal to 2/3 of pi times the radius raised to the third power.
In other words, when the height is equal to the diameter. The relation between radius, height and Volume changes completely.