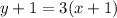Question

The given line passes through the points (0, -3) and (2, 3).

What is the equation, in point-slope form of the line that is
parallel to the given line and passes through the point
-1, - 1)?
y+1=3(x+1)
y+1=-=(x + 1)
-
532
v+1={(x+1)
y+1 =3(x+1)
Mark this and return
Save and Exit
S

Answer 1

The answer is: y+1=(3x+1)

Explanation:

Answer 2

`y+1=3(x+1)`

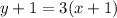
Explanation:

Ok so we are looking for line parallel to the line containing points (0,-3) and (2,3).

Parallel lines have the same slope.

So let's find the slope of the line containing the points (0,-3) and (2,3).

You can use the formula
`m=(y_2-y_1)/(x_2-x_1)`.

However, I just like to line up the points vertically and subtract them vertically, then put 2nd difference over 1st difference. Like this:

(0 , -3)

-(2 , 3)

-----------

-2 -6

So the slope is -6/-2 or just 3.

So the slope of the line we are looking for has slope 3 (or m=3) and your line should contain the point (-1,-1).

The point slope form of a line is:

`y-y_1=m(x-x_1)` where
`m` is the slope and
`(x_1,y_1)` is a point you know on the line.

So we just plug into that equation now. That gives us:

`y-(-1)=3(x-(-1))`

Simplify a bit:

`y+1=3(x+1)`