Question

Suppose that a department contains 9 men and 15 women. How many different committees of 6 members are possible if the committee must have strictly more women than men?

Answer 1

Answer: The required number of different possible committees is 81172.

Step-by-step explanation: Given that a department contains 9 men and 15 women.

We are to find the number of different committees of 6 members that are possible if the committee must have strictly more women than men.

Since we need committees of 6 members, so the possible combinations are

(4 women, 2 men), (5 women, 1 men) and (6 women).

Therefore, the number of different committees of 6 members is given by

`n\\\\\\=^(15)C_4* ^9C_2+^(15)C_5* ^9C_1+^(15)C_6\\\\\\=(15!)/(4!(15-4)!)* (9!)/(2!(9-2)!)+(15!)/(5!(15-5)!)* (9!)/(1!(9-1)!)+(15!)/(6!(15-6)!)\\\\\\\\=(15*14*13*12*11!)/(4*3*2*1*11!)*(9*8*7!)/(2*1*7!)+(15*14*13*12*11*10!)/(5*4*3*2*1*10!)*(9*8!)/(1*8!)+(15*14*13*12*11*10*9!)/(6*5*4*3*2*1*9!)\\\\\\=1365*36+3003*9+5005\\\\=49140+27027+5005\\\\=81172.`

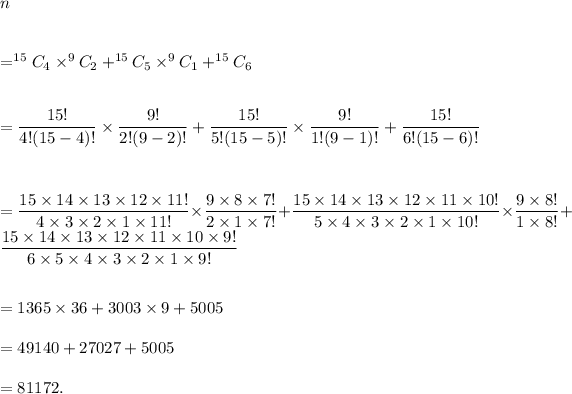
Thus, the required number of different possible committees of 6 members is 81172.