Question

A coin sold at auction in 2017 for $9,786,000. The coin had a face value of $15 when it was issued in 1794 and had previously been sold for $140,000 in 1973. a. At what annual rate did the coin appreciate from its first minting to the 1973 sale? (Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.) b. What annual rate did the 1973 buyer earn on his purchase? (Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.) c. At what annual rate did the coin appreciate from its first minting to the 2017 sale? (Do not round intermediate calculations and enter your answer as a percent rounded to 2 decimal places, e.g., 32.16.)

Answer 1

(A) 5.24%

(B) 10.13%

Step-by-step explanation:

To solve this we are going to use the the formula for compound interest

`$$Principal * (1 + rate)^(time) =$ Ammount`

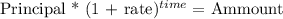
We are going to post the know values to get the rate

time = 1,973-1,794 = 179

`15 * (1 + rate)^(223) =$ 140,000 = 1.052395485`

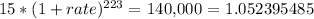
rate = 5.2395%

and for the second period we are going to do the same

time = 2017 - 1973 = 44

`140,000 * (1 + rate)^(44) =$ 9,786,000 = 1.101336254`

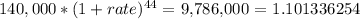
rate = 10.1336%