Question

Can someone please do 41 and 45???? Thanks!!!

Answer 1

Part 41) The solution of the compound inequality is equal to the interval [-1.5,-0.5)

Part 45) The solution of the compound inequality is equal to the interval

(-∞, -0.5] ∪ [1,∞)

Explanation:

Part 41) we have

`-4\leq 2+4x < 0`

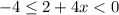
Divide the compound inequality into two inequalities

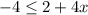`-4\leq 2+4x` -----> inequality A

Solve for x

Subtract 2 both sides

`-4-2\leq 4x`

`-6\leq 4x`

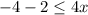
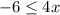
Divide by 4 both sides

`-1.5\leq x`

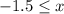
Rewrite

`x\geq -1.5`

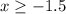
The solution of the inequality A is the interval -----> [-1.5,∞)

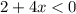`2+4x < 0` -----> inequality B

Solve for x

Subtract 2 both sides

`4x < -2`

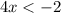
Divide by 4 both sides

`x < -0.5`

The solution of the inequality B is the interval ------> (-∞, -0.5)

The solution of the inequality A and the Inequality B is equal to

[-1.5,∞)∩ (-∞, -0.5)------> [-1.5,-0.5)

see the attached figure N 1

Part 45) we have

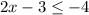`2x-3\leq -4` or
`3x+1\geq 4`

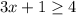
Solve the inequality A

`2x-3\leq -4`

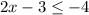
Adds 3 both sides

`2x\leq -4+3`

`2x\leq -1`

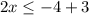
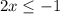
Divide by 2 both sides

`x\leq -0.5`

The solution of the inequality A is the interval ------> (-∞, -0.5]

Solve the inequality B

`3x+1\geq 4`

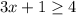
Subtract 1 both sides

`3x\geq 4-1`

`3x\geq 3`

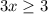
Divide by 3 both sides

`x\geq 1`

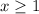
The solution of the inequality B is the interval -----> [1,∞)

The solution of the compound inequality is equal to

(-∞, -0.5] ∪ [1,∞)

see the attached figure N 2