Answer:
Option C. x = -2,6 extreme value at (2.16)
Explanation:
we have
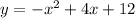
This is the equation of a vertical parabola open down
The vertex is a maximum (extreme value)
Convert the equation into vertex form
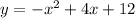
Complete the square
Group terms that contain the same variable and move the constant term to the left side

Factor -1

Remember to balance the equation by adding the same constants to each side.
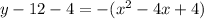
Rewrite as perfect squares
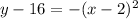
 -----> equation of the parabola in vertex form
-----> equation of the parabola in vertex form
The vertex is the point (2,16) ----> is a maximum (extreme value)
Determine the solutions of the quadratic equation
For y=0

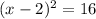
square root both sides
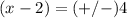

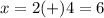

therefore
The solutions are x=-2 and x=6
The extreme value is (2,16)