Answer:
The discriminant of the equation is zero.
Explanation:
The given graph is a quadratic equation. If x = 6 is the only x-intercept of the graph, then the roots must be equal.
The quadratic equation will have two solutions. Here the two solutions are equal x = 6.
If the roots are equal, then the discriminant is zero.
The factors of the quadratic equation (x - 6) (x - 6)
=
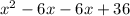
=
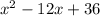
Discriminant =
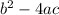
Here a = 1, b = -12 and c = 36
Discriminant =

= 144 - 144
= 0
Therefore, the answer is "The discriminant of the equation is zero."