Question

PLEASE HELP

A rectangular pyramid has a height of 6 units and a volume of 40 units3. Shannon states that a rectangular prism with the same base area and height has a volume that is three times the size of the given rectangular pyramid. Which statement explains whether Shannon is correct?

A rectangular prism in which BA = 20 and h = 6 has a volume of 40 units3; therefore, Shannon is incorrect.

A rectangular prism in which BA = 6.67 and h = 6 has a volume of 40 units3; therefore, Shannon is incorrect.

A rectangular prism in which BA = 20 and h = 6 has a volume of 120 units3; therefore, Shannon is correct.

A rectangular prism in which BA = 6.67 and h = 6 has a volume of 120 units3; therefore, Shannon is correct.

Answer 1

C

Explanation:

Got it right one the test! <3

Answer 2

A rectangular prism in which BA = 20 and h = 6 has a volume of 120 units3; therefore, Shannon is correct

Explanation:

step 1

Find the area of the base of the rectangular pyramid

we know that

The volume of the rectangular pyramid is equal to

`V=(1)/(3)BH`

where

B is the area of the base

H is the height of the pyramid

we have

`V=40\ units^(3)`

`H=6\ units`

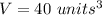
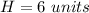
substitute and solve for B

`40=(1)/(3)B(6)`

`120=B(6)`

`B=120/6=20\ units^(2)`

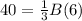
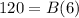
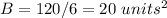
step 2

Find the volume of the rectangular prism with the same base area and height

we know that

The volume of the rectangular prism is equal to

`V=BH`

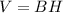
we have

`B=20\ units^(2)`

`H=6\ units`

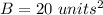
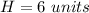
substitute

`V=(20)(6)=120\ units^(3)`

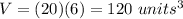
therefore

The rectangular prism has a volume that is three times the size of the given rectangular pyramid. Shannon is correct