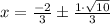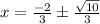Question

If the quadratic formula is used to find the solution set of 3x2 + 4x - 2 = 0, what are the solutions?

Answer 1

`x=(-2)/(3) \pm (√(10))/(3)`

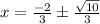
Explanation:

Compare
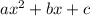`ax^2+bx+c` to
`3x^2+4x-2`.

We have
`a=3,b=4,c=-2`.

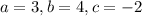The quadratic formula is for solving equations of the form
`ax^2+bx+c=0` and is
`x=(-b \pm √(b^2-4ac))/(2a)`.

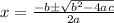So we are going to plug in our values in that formula to find our solutions,x.

If you want to notice it in parts you can.

Example I might break it into these parts and then put it in:

Part 1: Evaluate
`b^2-4ac`

Part 2: Evaluate
`-b`

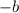
Part 3: Evaluate
`2a`

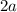
------Let's do these parts.

Part 1:
`b^2-4ac=(4)^2-4(3)(-2)=16-12(-2)=16+24=40`.

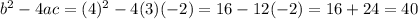This part 1 is important in determining the kinds of solutions you have. It is called the discriminant. If it is positive, you have two real solutions. If it is negative, you have no real solutions (both of the solutions are complex). If it is 0, you have one real solution.

Part 2:
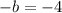`-b=-4` since
`b=4`.

Part 3:
`2a=2(3)=6`.

Let's plug this in:

`x=(-b \pm √(b^2-4ac))/(2a)`

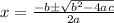
or in terms of our parts:

`x=\frac{\text{Part 2} \pm \sqrt{\text{Part 1}}}{\text{Part 3}}`

`x=(-4 \pm √(40))/(6)`

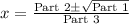
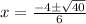
40 itself is not a perfect square but it does contain a factor that is. That factor is 4.

So we are going to rewrite 40 as
`4 \cdot 10`.

`x=(-4 \pm √(4 \cdot 10))/(6)`

`x=(-4 \pm √(4) \cdot √(10))/(6)`

`x=(-4 \pm 2\cdot √(10))/(6)`

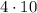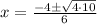
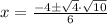
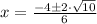
I'm going to go ahead and separate the fraction like so:

`x=(-4)/(6) \pm (2 \cdot √(10))/(6)`

Now I'm going to reduce both fractions:

`x=(-2)/(3) \pm (1 \cdot √(10))/(3)`

`x=(-2)/(3) \pm (√(10))/(3)`