Question

Using a graphical approach, to determine the type of the problem,suggest a strategy to avoid the problem( if any), maximize 10X+10Y subject to : 2X+4Y=< 16 2X=<10

4Y=<8 X=6

Answer 1

No solution. Inconsistent.

Explanation:

The equations are

`2x+4y\leq 16\\x\leq 5\\y\leq 2\\x=6`

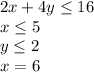
As we can see here that x=6 and x<=5 the solution area will not be bounded i.e., there will be no common area.

The lines do not intersect

Therefore there will be no solution.

Plotting the equations we get the graph below.

A strategy to avoid the question would be by just looking at the linear equations. It can be clearly seen that there are two lines that will never intersect and hence will have no solution.