Question

find the coordinates of P so that P partitions the segment AB in the ratio 1:1 is A(-4,15) and B(10,11)

Answer 1

(3,13)

Explanation:

I got it correct on founders edtell

Answer 2

The coordinates of point P are (3 , 13)

Explanation:

* Lets explain how to solve the problem

- Point P divides the segment AB in the ratio 1 : 1

- The ratio 1 : 1 means divide the segment into two equal parts

- Then P is the mid-point of segment AB

- If (x , y) are the coordinates of the mid-point of a segments whose

endpoints are (x1 , y1) and (x2 , y2) then;

`x=(x_(1)+x_(2))/(2),y=(y_(1)+y_(2))/(2)`

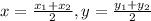
∵ The coordinates of point A is (-4 , 15)

∵ The coordinates of point B is 10 , 11)

- Let point A is (x1 , y1) , point B is (x2 , y2) and point P is (x , y)

∵ x1 = -4 , x2 = 10 and y1 = 15 , y2 = 11

∴
`x=(-4+10)/(2)=(6)/(2)=3`

∴
`y=(15+11)/(2)=(26)/(2)=13`

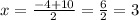
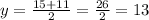
∴ The coordinates of point P are (3 , 13)