Question

Verify that y = c_1 + c_2 e^2x is a solution of the ODE y" - 2y' = 0 for all values of c_1 and c_2.

Answer 1

For any value of C1 and C2,
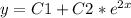`y = C1 + C2*e^(2x)` is a solution.

Explanation:

Let's verify the solution, but first, let's find the first and second derivatives of the given solution:

`y = C1 + C2*e^(2x)`

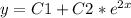
For the first derivative we have:

`y' = 0 + C2*(2x)'*e^(2x)`

`y' = C2*(2)*e^(2x)`

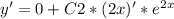
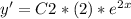
For the second derivative we have:

`y'' = C2*(2)*(2x)'*e^(2x)`

`y'' = C2*(2)*(2)*e^(2x)`

`y'' = C2*(4)*e^(2x)`

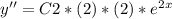
Let's solve the ODE by the above equations:

`y'' - 2y' = 0`

`C2*(4)*e^(2x) - 2*C2*(2)*e^(2x) = 0`

`C2*(4)*e^(2x) - C2*(4)*e^(2x) = 0`

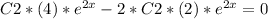
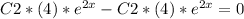
From the above equation we can observe that for any value of C2 the equation is solved, and because the ODE only involves first (y') and second (y'') derivatives, C1 can be any value as well, because it does not change the final result.