Question

Please help ASAP !

Consider the quadratic function f(x)=x^2−5x−6.



Determine the following: (enter all numerical answers as integers, fractions, or decimals):



The smallest x-intercept is x=____ .



The largest x-intercept is x=____ .



The y-intercept is y=_____ .



The vertex is ( ___ , ___ ).



The line of symmetry has the equation _____.

Answer 1

Part 1) The smallest x-intercept is x=-1

Part 2) The largest x-intercept is x=6

Part 3) The y-intercept is y=-6

Part 4) The vertex is the point (2.5,-12.25)

Part 5) The equation of the line of symmetry is x=2.5

Explanation:

we have

`f(x)=x^(2)-5x-6`

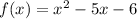
step 1

Find the x-intercepts

we know that

The x-intercept is the value of x when the value of the function is equal to zero

so

equate the function to zero

`x^(2)-5x-6=0`

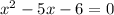
The formula to solve a quadratic equation of the form
`ax^(2) +bx+c=0` is equal to

`x=\frac{-b(+/-)\sqrt{b^(2)-4ac}} {2a}`

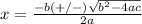
in this problem we have

`x^(2)-5x-6=0`

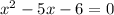
so

`a=1\\b=-5\\c=-6`

substitute in the formula

`x=\frac{-(-5)(+/-)\sqrt{-5^(2)-4(1)(-6)}} {2(1)}`

`x=\frac{5(+/-)√(49)} {2}`

`x=\frac{5(+/-)7} {2}`

`x=\frac{5(+)7} {2}=6`

`x=\frac{5(-)7} {2}=-1`

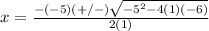
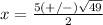
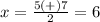
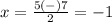
therefore

The x-intercepts are

x=-1 and x=6

The smallest x-intercept is x=-1

The largest x-intercept is x=6

step 2

Find the y-intercept

we know that

The y-intercept is the value of y when the value of x is equal to zero

so

For x=0

`f(0)=(0)^(2)-5(0)-6`

`f(0)=-6`

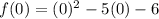
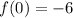
therefore

The y-intercept is y=-6

step 3

Find the vertex

we know that

The equation of a vertical parabola into vertex form is equal to

`f(x)=a(x-h)^(2)+k`

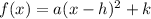
where

(h,k) is the vertex

Convert the function into vertex form

`f(x)=x^(2)-5x-6`

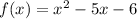
Group terms that contain the same variable, and move the constant to the opposite side of the equation

`f(x)+6=(x^(2)-5x)`

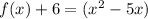
Complete the square, Remember to balance the equation by adding the same constants to each side

`f(x)+6+2.5^(2)=(x^(2)-5x+2.5^(2))`

`f(x)+12.25=(x^(2)-5x+6.25)`

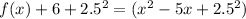
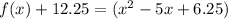
Rewrite as perfect squares

`f(x)+12.25=(x-2.5)^(2)`

`f(x)=(x-2.5)^(2)-12.25`

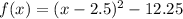
The vertex is the point (2.5,-12.25)

step 4

Find the equation of the line of symmetry

we know that

In a vertical parabola the equation of the line of symmetry is equal to the x-coordinate of the vertex

we have

vertex (2.5,-12.25)

The x-coordinate of the vertex is 2.5

therefore

The equation of the line of symmetry is x=2.5