Answer:
No real solutions.
Explanation:
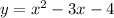
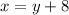
I'm going to subtract the second expression for y and plug it into the first equation.
So solving x=y+8 for y by subtracting 8 on both sides gives us y=x-8.
I'm going to insert this for the first y like so:
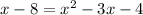
Now I'm going to move everything to one side.
I'm going to subtract x on both sides and add 8 on both sides.

Simplifying:

Now our job since the coefficient of x^2 is 1 is to find two numbers that multiply to be 4 and at the same time add up to be -3. I can't think of any such numbers.
Let's check the discriminant.
Compare
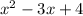 to
to
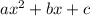 .
.
So
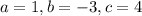 .
.
The discriminant is
 .
.
So plugging in our numbers we get
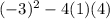 .
.
Time to simplify:
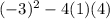

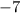
So since the discriminant is negative, then the solutions will not be real.