Answer:
Safe load of the beam is 3000 pounds.
Explanation:
If a horizontal beam of x feet length can hold y pounds safe load, the expression that represents the relation between load and length of the beam is
y =

If y = 2000 pounds and x = 15 feet
then 2000 =
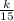
k = 15×2000 = 30000
Now we will calculate the safe load when beam is 10 feet long.
From the formula,
y =
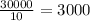 pounds
pounds
Therefore, safe load of the beam is 3000 pounds.