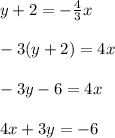2 Answers

Ali Tourani · Answer 1 · 2020-09-19T17:14:59+0000

Answer: last option.

Explanation:

The equation of the line in Slope-Intercept form is:

y=mx+b

Where "m" is the slope and "b" is the y-intercept.

Knowing that the given line passes through the points (0,3) and (3,-1), we can find the slope:

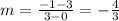
m=(-1-3)/(3-0)=-(4)/(3)

Since the other line is parallel to this line, its slope must be equal:

m=-(4)/(3)

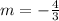
Substitute the slope and the point (-3, 2) into
y=mx+b and solve for "b":

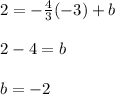
$2=-(4)/(3)(-3)+b\\\\2-4=b\\\\b=-2$

Then, the equation of the other line in Slope-Intercept form is:

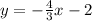
y=-(4)/(3)x-2

Rewriting it in Standard form, you get:

$y+2=-(4)/(3)x\\\\-3(y+2)=4x\\\\-3y-6=4x\\\\4x+3y=-6$

Tamriel · Answer 2 · 2020-09-20T16:07:31+0000

For this case we have that by definition, if two lines are parallel their slopes are equal.

The line given for the following points:

(0,3) and (3, -1). Then the slope is:

$m = \frac {y2-y1} {x2-x1} = \frac {-1-3} {3-0} = \frac {-4} {3} = - \frac {4} {3}$

Then, the requested line will be of the form:

$y = - \frac {4} {3} x + b$

To find "b" we substitute the given point:

$2 = - \frac {4} {3} (- 3) + b\\2 = 4 + b\\2-4 = b\\b = -2$

Finally, the line is:

$y = - \frac {4} {3} x-2$

By manipulating algebraically we have:

$y + 2 = - \frac {4} {3} x\\3 (y + 2) = - 4x\\3y + 6 = -4x\\4x + 3y = -6$

Answer:

Option D

Please log in or register to add a comment.