Answer:
Last option: Translate each point of the graph of h(x) 3 units left.
Explanation:
There are some transformations for a function f(x). The following is one of these transformations:
If
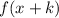 , then the function is shifted "k" units to the left.
, then the function is shifted "k" units to the left.
Given the function
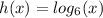 and the function
and the function
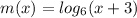 , you can notice that the function m(x) is the function h(x) but shifted left 3 units.
, you can notice that the function m(x) is the function h(x) but shifted left 3 units.
Therefore, you could graph the function m(x) by translating each point of the graph of the function h(x) 3 units left.
This matches with the last option.