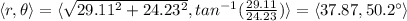Initially, the velocity vector is
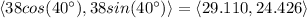 . At the same height, the x-value of the vector will be the same, and the y-value will be opposite (assuming no air resistance). Assuming perfect reflection off the ground, the velocity vector is the same. After 0.2 seconds at 9.8 seconds, the y-value has decreased by
. At the same height, the x-value of the vector will be the same, and the y-value will be opposite (assuming no air resistance). Assuming perfect reflection off the ground, the velocity vector is the same. After 0.2 seconds at 9.8 seconds, the y-value has decreased by
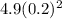 , so the velocity is
, so the velocity is
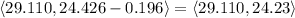 .
.
Converting back to direction and magnitude, we get