Question

For a standard normal distribution find the approximate value of p(z<0.42)

Answer 1

0.66276.

Explanation:

We are asked to find the approximate value of p(z<0.42) for a standard normal distribution.

Our given expression means the probability of getting a z-score less than 0.42.

We need to find the probability of getting the area corresponding to a z-score less than 0.42 under normal distribution curve.

We will normal distribution table to solve our given problem.

`p(z<0.42)=0.66276`

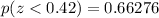
Therefore, the approximate value of our given expression would be 0.66276.

Answer 2

p(z<0.42) = 0.6628

Explanation:

A normal distribution with a mean of 0 and a standard deviation of 1 is called a standard normal distribution. That is to say:

μ = 0

σ² = 1

Using a calculator, we find that:

p(z<0.42) = 0.6628 (See picture attached)