Question

What is the percent yield of a reaction in which 74.1 g of tungsten(VI) oxide (WO3) reacts with excess hydrogen gas to produce metallic tungsten and 8.01 mL of water (d = 1.00 g/mL)?

Answer 1

Answer : The percent yield of the reaction is, 46.49 %

Explanation : Given,

Mass of
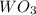`WO_3` = 74.1 g

Molar mass of
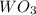`WO_3` = 231.84 g/mole

Molar mass of
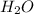`H_2O` = 18 g/mole

First we have to calculate the moles of
`WO_3`.

`\text{Moles of }WO_3=\frac{\text{Mass of }WO_3}{\text{Molar mass of }WO_3}=(74.1g)/(231.84g/mole)=0.319mole`

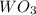Now we have to calculate the moles of
`H_2O`.

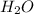The balanced chemical reaction will be,

`WO_3+3H_2\rightarrow W+3H_2O`

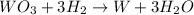
From the balanced reaction, we conclude that

As, 1 mole of
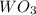`WO_3` react to give 3 moles of
`H_2O`

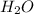
So, 0.319 moles of
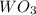`WO_3` react to give
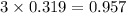`3* 0.319=0.957` moles of
`H_2O`

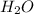
Now we have to calculate the mass of
`H_2O`

`\text{Mass of }H_2O=\text{Moles of }H_2O* \text{Molar mass of }H_2O`

`\text{Mass of }H_2O=(0.957mole)* (18g/mole)=17.226g`

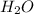
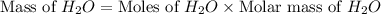
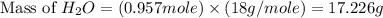
The theoretical yield of
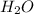`H_2O` = 17.226 g

Now we have to calculate the actual yield of water.

`\text{Actual mass of water}=\text{Density of water}* \text{Volume of water}=1.00g/ml* 8.01ml=8.01g`

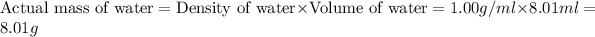
The actual yield of
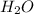`H_2O` = 8.01 g

Now we have to calculate the percent yield of
`H_2O`

`\%\text{ yield of }H_2O=\frac{\text{Actual yield of }H_2O}{\text{Theoretical yield of }H_2O}* 100=(8.01g)/(17.226g)* 100=46.49\%`

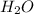
Therefore, the percent yield of the reaction is, 46.49 %