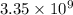Question

Two spherical objects with a mass of 6.22 kg each are placed at a distance of 1.02 m apart. How many electrons need to leave each object so that the net force between them becomes zero?

Answer 1

The number of electrons need to leave each object is
`3.35*10^(9)`

Step-by-step explanation:

Given that,

Mass of object = 6.22 kg

Distance = 1.02 m

We need to calculate the number of electron

Using formula of electric force

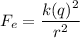`F_(e)=(k(q)^2)/(r^2)`....(I)

We know that,

`q = Ne`

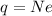
Put the value of q in equation (I)

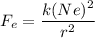`F_(e)=(k(Ne)^2)/(r^2)`.....(II)

Using gravitational force

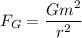`F_(G)=(Gm^2)/(r^2)`.....(III)

Equating equation (II) and (III)

`F_(e)=F_(G)`

`(k(Ne)^2)/(r^2)=(Gm^2)/(r^2)`

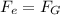
`N=\sqrt{(G)/(k)}*(m)/(e)`....(IV)

Put the value in the equation(IV)

`N=\sqrt{(6.67*10^(-11))/(9*10^(9))}*(6.22)/(1.6*10^(-19))`

`N=3.35*10^(9)`

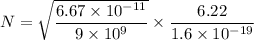
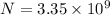
Hence, The number of electrons need to leave each object is
`3.35*10^(9)`