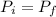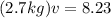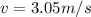Question

A 2.7-kg cart is rolling along a frictionless, horizontal track towards a 1.1-kg cart that is held initially at rest. The carts are loaded with strong magnets that cause them to attract one another. Thus, the speed of each cart increases. At a certain instant before the carts collide, the first cart's velocity is +3.7 m/s, and the second cart's velocity is -1.6 m/s. (a) What is the total momentum of the system of the two carts at this instant? (b) What was the velocity of the first cart when the second cart was still at rest?

Answer 1

Part a)

P = 8.23 kg m/s

Part b)

v = 3.05 m/s

Step-by-step explanation:

Part a)

momentum of cart 1 is given as

`P_1 = m_1v_1`

`P_1 = (2.7)(3.7) = 9.99 kg m/s`

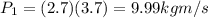
Momentum of cart 2 is given as

`P_2 = m_2v_2`

`P_2 = (1.1)(-1.6) = -1.76 kg m/s`

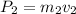
Now total momentum of both carts is given as

`P = P_1 + P_2`

`P = 8.23 kg m/s`

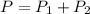
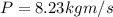
Part b)

Since two carts are moving towards each other due to mutual attraction force and there is no external force on two carts so here momentum is always conserved

so here we will have

`P_i = P_f`

`(2.7 kg)v = 8.23`

`v = 3.05 m/s`