Question

Stackable polystyrene cups have a height h1=12.5 cm. Two stacked cups have a height of h2=14 cm. Three stacked cups have a height of h3=15.5 cm. Find the equation for hx= 1.5 x+ Your friend is 200 cm tall. Find out how many cups you will need to reach the height of your friend. cups

Answer 1

hx = 1.5cm . x + 11 cm

126 cups

Explanation:

We have the following ordered pairs (x, hx).

• (1, 12.5 cm)

• (2, 14 cm)

• (3, 15.5 cm)

We are looking for a linear equation of the form:

hx = a.x + b

where,

a is the slope

b is the y-intercept

To find the slope, we take any pair of ordered values and replace their values in the following expression.

`a=(\Delta hx )/(\Delta x) =(h2-h1)/(2-1) =(14cm-12.5cm)/(2-1) =1.5cm`

Now, the general form is:

hx = 1.5cm . x + b

We can take any ordered pair and replace it in this expression to find b. Let's use h1.

h1 = 1.5cm . x1 + b

12.5 cm = 1.5 cm . 1 + b

b = 11 cm

The final equation is:

hx = 1.5cm . x + 11 cm

If hx = 200 cm,

200 cm = 1.5cm . x + 11 cm

189 cm = 1.5cm . x

x = 126

Answer 2

Approximately 59 stacked cups.

Explanation:

Given,

Height of a cup = 12.5 cm,

Two stacked cups = 14 cm,

Three stacked cups = 15.5 cm,

........, so on,....

Thus, there is an AP that represents the given situation,

12.5, 14, 15.5,....

First term is, a = 12.5,

Common difference, d = 1.5 cm,

Thus, the height of x cups is,

`h(x) = a+(x-1)d = 12.5 + (x-1)1.5 = 1.5x + 11`

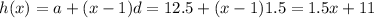
According to the question,

h(x) = 200

⇒ 1.5x + 11 = 200

⇒ 1.5x = 189

⇒ x = 59.3333333333 ≈ 59,

Hence, approximately 59 stacked cups will need.