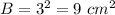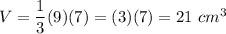Answer:

Explanation:
4.
The formula of a volume of a cone:
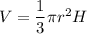
r - radius
H - height
We have

Substitute:
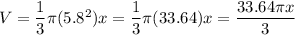

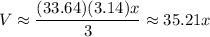
5.
The formula of a volume of a pyramid:
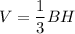
B - base area
H - height
In the base we have the square. The formula of an area of a square with side s:

We have