Question

Suppose that a company's annual sales were $1,200,000 in 1999. The annual growth rate of sales from 1999 to 2000 was 16 percent, from 2000 to 2001 it was −5 percent, and from 2001 to 2002 it was 22 percent.

The geometric mean growth rate of sales over this three-year period is calculated as 10.37 percent. Use the geometric mean growth rate and determine the forecasted sales for 2004.

Answer 1

Explanation:

A = P (1 + r)^t

Given that P = $1,200,000, r = 0.1037, and t = 5:

A = $1,200,000 (1 + 0.1037)^5

A = $1,965,334.41

Round as needed.

Answer 2

The forecasted sales for 2004 is $1965281.

Explanation:

The annual sales in 1999 were = $1,200,000

Let geometric mean growth rate = r

we have now p = $1,200,000,

r = 10.37% or 0.1037

t = 5:

We have the formula Amt=
`p(1+r)^(t)`

Amt =
`1200000(1+0.1037)^(5)`

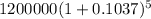
Solving this we get;

`1200000*1.63777=1965324`

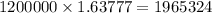
A = $1,965,324