Question

The third term of an arithmetic progression is 6 while the sum of the first twelve terms is 282. Find the common difference and the first term​

Answer 1

d = 5 and a₁ = - 4

Explanation:

The n th term of an arithmetic sequence is

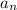`a_(n)` = a₁ + (n - 1)d

Given a₃ = 6, then

a₁ + 2d = 6 → (1)

The sum to n terms of an arithmetic sequence is

`S_(n)` =
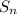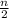`(n)/(2)` [ 2a₁ + (n - 1)d ]

Given
`S_(12)` = 282, then

6 [ 2a₁ + 11d ] = 282 ( divide both sides by 6 )

2a₁ + 11d = 47 → (2)

We can now solve (1) and (2) for d and a₁

Multiply (1) by - 2

- 2a₁ - 4d = - 12 → (3)

Add (2) and (3) term by term

7d = 35 ( divide both sides by 7 )

d = 5

Substitute d = 5 in (1) and solve for a₁

a₁ + 10 = 6 ( subtract 10 from both sides )

a₁ = - 4