Question

Write an equation in standard form for each ellipse with center (0, 0) and co-vertex at (5, 0); focus at (0, 3).

Answer 1

The required standard form of ellipse is
`(x^2)/(25)+(y^2)/(34)=1`.

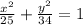Explanation:

The standard form of an ellipse is

`((x-h)^2)/(a^2)+((y-k)^2)/(b^2)=1`

Where, (h,k) is center of the ellipse.

It is given that the center of the circle is (0,0), so the standard form of the ellipse is

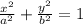`(x^2)/(a^2)+(y^2)/(b^2)=1` .... (1)

If a>b, then coordinates of vertices are (±a,0), coordinates of co-vertices are (0,±b) and focus (±c,0).

`c^2=a^2-b^2` .... (2)

If a<b, then coordinates of vertices are (0,±b), coordinates of co-vertices are (±a,0) and focus (0,±c).

`c^2=b^2-a^2` .... (3)

It is given that co-vertex of the ellipse at (5, 0); focus at (0, 3). So, a<b we get

`a=5,c=3`

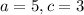
Substitute a=5 and c=3 these values in equation (3).

`3^2=b^2-(5)^2`

`9=b^2-25`

`34=b^2`

`√(34)=b`

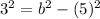
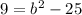
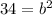
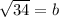
Substitute a=5 and
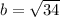`b=√(34)` in equation (1), to find the required equation.

`(x^2)/(5^2)+(y^2)/((√(34))^2)=1`

`(x^2)/(25)+(y^2)/(34)=1`

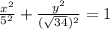
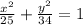
Therefore the required standard form of ellipse is
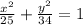`(x^2)/(25)+(y^2)/(34)=1`.