Question

In a circus performance, a large 3.0 kg hoop with a radius of 1.3 m rolls without slipping. If the hoop is given an angular speed of 6.8 rad/s while rolling on the horizontal and is allowed to roll up a ramp inclined at 24◦ with the horizontal, how far (measured along the incline) does the hoop roll? The acceleration of gravity is 9.81 m/s2 .

Answer 1

The distance is 19.58 m.

Step-by-step explanation:

Given that,

Mass = 3.0 kg

Radius = 1.3 m

Angular speed = 6.8 rad/s

Angle = 24°

Acceleration of gravity = 9.81 m/s²

We need to calculate the distance

Using formula of kinetic energy

Total Initial kinetic energy,

`K.E = (1)/(2)(mv^2+I\omega^2)`

`K.E = (1)/(2)(mv^2+mr^2\omega^2)`

`K.E =((mr^2+mr^2)\omega^2)/(2)`

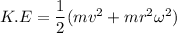
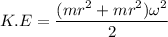
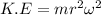`K.E =mr^2\omega^2`....(I)

Now, Total potential energy

`P.E=mgs\sin\theta`

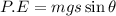
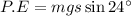`P.E=mgs\sin24^(\circ)`....(II)

Equating equation (I) and (II)

`mr^2\omega^2==mgs\sin24^(\circ)`

`(1.3)^2*6.8^2=9.81* s*\sin24^(\circ)`

`s = (1.3^2*6.8^2)/(9.81*\sin24^(\circ))`

`s=19.58\ m`

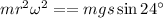
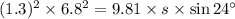
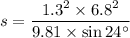
Hence, The distance is 19.58 m.