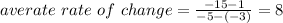Answer: OPTION C.
Explanation:
You need to use this formula:
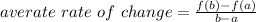
Knowing that we need to find the average rate of change for the given quadratic function, for the interval from
 to
to
 , we need to find their corresponding y-coordinates.
, we need to find their corresponding y-coordinates.
We can observe in the graph that:
For
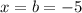 →
→
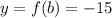
For
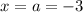 →
→

Therefore, substitituting, we get: