Question

A rigid vessel contains 3.98 kg of refrigerant-134a at 700 kPa and 60°C. Determine the volume of the vessel and the total internal energy. m3 (Round to four decimal places) kJ (Round to one decimal place)

Answer 1

Answer: The volume of the vessel is
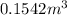`0.1542m^3` and total internal energy is 162.0 kJ.

Step-by-step explanation:

• To calculate the volume of water, we use the equation given by ideal gas, which is:

`PV=nRT`

or,

`PV=(m)/(M)RT`

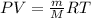
where,

P = pressure of container = 700 kPa

V = volume of container = ? L

m = Given mass of R-134a = 3.98 kg = 3980 g (Conversion factor: 1kg = 1000 g)

M = Molar mass of R-134a = 102.03 g/mol

R = Gas constant =
`8.31\text{L kPa }mol^(-1)K^(-1)`

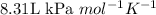
T = temperature of container =
`60^oC=[60+273]K=333K`

Putting values in above equation, we get:

`700kPa* V=(3980g)/(102.03g/mol)* 8.31\text{L kPa }* 333K\\\\V=154.21L`

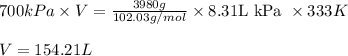
Converting this value into
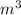`m^3`, we use the conversion factor:

`1m^3=1000L`

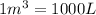
So,
`\Rightarrow ((1m^3)/(1000L))* 154.21L`

`\Rightarrow 0.1542m^3`

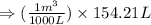
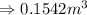
• To calculate the internal energy, we use the equation:

`U=(3)/(2)nRT`

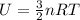
or,

`U=(3)/(2)(m)/(M)RT`

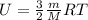
where,

U = total internal energy

m = given mass of R-134a = 3.98 kg = 3980 g (Conversion factor: 1kg = 1000g)

M = molar mass of R-134a = 102.03 g/mol

R = Gas constant =
`8.314J/K.mol`

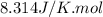
T = temperature =
`60^oC=[60+273]K=333K`

Putting values in above equation, we get:

`U=(3)/(2)* (3980g)/(102.03g/mol)* 8.314J/K.mol* 333K\\\\U=161994.6J`

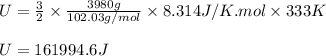
Converting this into kilo joules, we use the conversion factor:

1 kJ = 1000 J

So, 161994.6 J = 162.0 kJ

Hence, the volume of the vessel is
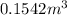`0.1542m^3` and total internal energy is 162.0 kJ.