Question

If A and B are two points in the plane , the perpendicular bisector of AB is the set of all points equidistant from A and B ? A True or False

Answer 1

The correct option is A. The given statement is true.

Explanation:

Given statement: If A and B are two points in the plane , the perpendicular bisector of AB is the set of all points equidistant from A and B.

Let a line is perpendicular bisector of AB at point D and C be a random point of perpendicular bisector.

In triangle ACD and BCD,

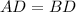`AD=BD` (Definition of perpendicular bisector)

`\angle ADC=\angle BDC` (Definition of perpendicular bisector)

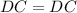`DC=DC` (Reflexive property)

By SAS postulate of congruence,

`\triangle ACD\cong \triangle BCD`

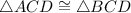
The corresponding parts of congruent triangles are congruent.

`AC\cong BC` (CPCTC)

`AC=BC`

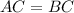
The distance between A to C and B to C are same. So, the set of all points on perpendicular bisector are equidistant from A and B.

The given statement is true. Therefore the correct option is A.