Answer: The molar mass of the gas is 35.87 g/mol.
Step-by-step explanation:
To calculate the mass of gas, we use the equation given by ideal gas:
PV = nRT
or,
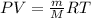
where,
P = Pressure of gas = 945 mmHg
V = Volume of the gas = 0.35 L
m = Mass of gas = 0.527 g
M = Molar mass of gas = ? g/mo
R = Gas constant =
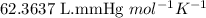
T = Temperature of gas =
![88^oC=[88+273]=361K](https://img.qammunity.org/2020/formulas/chemistry/college/am5qh6uaryt6862f1rcaakoh1sqb9xrnts.png)
Putting values in above equation, we get:
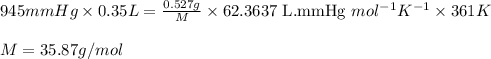
Hence, the molar mass of the gas is 35.87 g/mol.