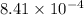Question

Methanol can be synthesized from monoxide and hydrogen gas at 525 K. A reaction mixture consisting initially of 1.8 moles of CO and 2.2 moles of H2 in 5.0-L container was found to contain 0.6 moles of CH3OH after reaching equilibrium (a) Calculate equilibrium concentration (in molarity) of CO and H2 (B) Calculate equilibrium constants kc and kp for this reaction

Answer 1

For a: The equilibrium concentration of CO and
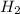`H_2` are 0.24 M and 0.32 M.

For b: The value of
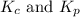`K_c\text{ and }K_p` are 1.5625 and
`8.41* 10^(-4)`

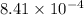
Step-by-step explanation:

We are given:

Volume of container = 5 L

Initial moles of CO = 1.8 moles

Initial concentration of CO =
`(1.8mol)/(5L)`

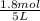
Initial moles of
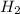`H_2` = 2.2 moles

Initial concentration of
`H_2` =
`(2.2mol)/(5L)`

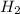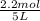
Equilibrium moles of
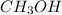`CH_3OH` = 0.6

Equilibrium concentration of
`CH_3OH` =
`(0.6mol)/(5L)=0.12M`

• For a:

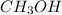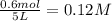
The chemical equation for the formation of methanol follows:

`CO(g)+H_2(g)\rightleftharpoons CH_3OH(l)`

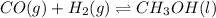
t = 0
`(1.8)/(5)`
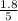
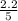`(2.2)/(5)` 0

`t=t_(eq)`
`(1.2)/(5)`
`(1.6)/(5)`
`(0.6)/(5)`

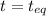
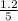
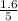
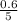
So, the equilibrium concentration of CO =
`(1.2)/(5)=0.24M`

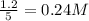
The equilibrium concentration of
`H_2` =
`(1.6)/(5)=0.32M`

• For b:

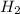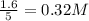
The expression of
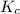`K_c` for the given chemical reaction follows:

`K_c=([CH_3OH])/([CO][H_2])`

We are given:

`[CH_3OH]=0.12mol/L`

`[CO]=0.24mol/L`

`[H_2]=0.32mol/L`

Putting values in above equation, we get:

`K_c=(0.12)/(0.24* 0.32)=1.5625`

Relation of
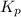`K_p` with
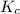`K_c` is given by the formula:

`K_p=K_c(RT)^(\Delta ng)`

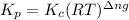
Where,

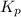`K_p` = equilibrium constant in terms of partial pressure = ?

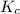`K_c` = equilibrium constant in terms of concentration = 1.5625

R = Gas constant =
`0.0821\text{ L atm }mol^(-1)K^(-1)`

T = temperature = 525 K

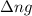`\Delta ng` = change in number of moles of gas particles =
`n_(products)-n_(reactants)=0-2=-2`

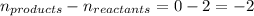
Putting values in above equation, we get:

`K_p=1.5625* (0.0821* 525)^(-2)\\\\K_p=8.41* 10^(-4)`

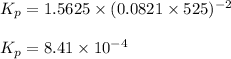
Hence, the value of
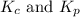`K_c\text{ and }K_p` are 1.5625 and
`8.41* 10^(-4)`