Question

Calculate the centripetal force on a 6.0 kg sensor that is attached at the end of a 100.0-m a long wind turbine blade that is rotating at 1.25 rev/s.

Answer 1

Centripetal force, F = 18486.75 N

Step-by-step explanation:

It is given that,

Mass of the sensor, m = 6 kg

It is attached at the end of 100 m long wind turbine, d = 100 m

So, the radius of the wind turbine, r = 50 m

Angular velocity,
`\omega=1.25\ rev/s=7.85\ rad/s`

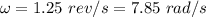
The centripetal force is given by :

`F=(mv^2)/(r)`

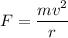
Since,
`v=r\omega`

`F=mr\omega^2`

`F=6\ kg* 50\ m* (7.85\ rad/s)^2`

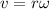
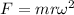
F = 18486.75 N

So, the centripetal force is 18486.75 N. Hence, this is the required solution.