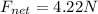Question

Charge 1 of +5 micro-coulombs is placed at the origin, charge 2 of +24 micro-coulombs is placed at x = +0.23 m, y = -0.69 m, charge 3 of -5 micro-coulombs is placed at x = -0.27 m, y = 0 m. What is the magnitude of the total electric force on charge 1 in Newtons?

Answer 1

`F_(net) = 4.22 N`

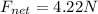
Step-by-step explanation:

Since charge 1 and charge 2 are positive in nature so here we will have repulsion type of force between them

It is given as

`F_(12) = (kq_1q_2)/(r^2)`

`F_(12) = ((9* 10^9)(5 \mu C)(24 \mu C))/(0.23^2 + 0.69^2)(-0.23\hat i + 0.69 \hat j)/(√(0.23^2 + 0.69^2))`

`F_(12) = 2.81(-0.23\hat i + 0.69\hat j)`

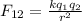
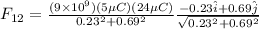
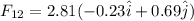
Since charge three is a negative charge so the force between charge 1 and charge 3 is attraction type of force

`F_(13) = ((9* 10^9)(5 \mu C)(5 \mu C))/(0.27^2 + 0^2) (-\hat i)`

`F_(13) = 3.1(- \hat i)`

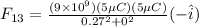
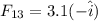
Now we will have net force on charge 1 as

`F_(net) = F_(12) + F_(13)`

`F_(net) = (-0.65 \hat i + 1.94 \hat j) + (-3.1 \hat i)`

`F_(net) = (-3.75 \hat i + 1.94 \hat j)`

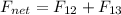
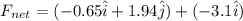
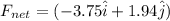
now magnitude of total force on the charge is given as

`F_(net) = 4.22 N`