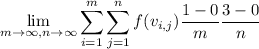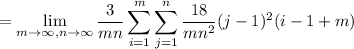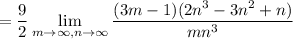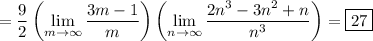Question

[15 points] Compute ffR2(x + 1)y2 dA, R = [ 0, 1] x [0,3), by Riemann sum definition. You must use the Riemann sum definition to receive credit.

Answer 1

Looks like the integral is

`\displaystyle\iint_R2(x+1)y^2\,\mathrm dA`

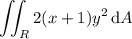
where
`R=[0,1]*[0,3]`. (The inclusion of
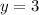`y=3` will have no effect on the value of the integral.)

Let's split up
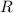`R` into
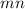`mn` equally-sized rectangular subintervals, and use the bottom-left vertices of each rectangle to approximate the integral. The intervals will be partitioned as

`[0,1]=\left[0,\frac1m\right]\cup\left[\frac1m,\frac2m\right]\cup\cdots\cup\left[\frac{m-1}m,1\right]`

and

`[0,3]=\left[0,\frac3n\right]\cup\left[\frac3n,\frac6n\right]\cup\cdots\cup\left[\frac{3(n-1)}n,3\right]`

where the bottom-left vertices of each rectangle are given by the sequence

`v_(i,j)=\left(\frac{i-1}n,\frac{3(j-1)}n\right)`

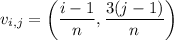
with
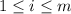`1\le i\le m` and
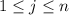`1\le j\le n`. Then the Riemann sum is

`\displaystyle\lim_(m\to\infty,n\to\infty)\sum_(i=1)^m\sum_(j=1)^nf(v_(i,j))\frac{1-0}m\frac{3-0}n`

`\displaystyle=\lim_(m\to\infty,n\to\infty)\frac3{mn}\sum_(i=1)^m\sum_(j=1)^n(18)/(mn^2)(j-1)^2(i-1+m)`

`\displaystyle=\lim_(m\to\infty,n\to\infty)(54)/(m^2n^3)\sum_(i=0)^(m-1)\sum_(j=0)^(n-1)j^2(i+m)`

`\displaystyle=\frac92\lim_(m\to\infty,n\to\infty)((3m-1)(2n^3-3n^2+n))/(mn^3)`

`\displaystyle=\frac92\left(\lim_(m\to\infty)\frac{3m-1}m\right)\left(\lim_(n\to\infty)(2n^3-3n^2+n)/(n^3)\right)=\boxed{27}`