Question

Find an equation for the line in the form ax + by c. where a. b. and c are integers with no factor common to all three and a 20. Through (1. -6), perpendicular to x + y = 2 The equation of the line is (Type an equation)

Answer 1

The standard form of required line is x-y=7.

Explanation:

The standard form of a line is

`ax+by=c`

Where, a,b,c are integers with no factor common to all three and a≥0.

The give equation of line is

`x+y=2`

Here a=1 and b=1.

The slope of a standard line is

`m=(-a)/(b)`

`m_1=(-1)/(1)=-1`

The product of slops of two perpendicular lines is -1.

`m_1\cdot m_2=-1`

`(-1)\cdot m_2=-1`

`m_2=1`

The slope of required line is 1.

The point slope form of a line is

`y-y_1=m(x-x_1)`

Where, m is slope.

The slope of required line is 1 and it passes through the point (1,-6). So, the equation of required line is

`y-(-6)=1(x-1)`

`y+6=x-1`

Add 1 on each side.

`y+7=x`

Subtract y from both the sides.

`7=x-y`

Therefore the standard form of required line is x-y=7.

Answer 2

a = 1, b = -1 and c = 7

Explanation:

Since, when two lines are parallel then the product of their slope is -1,

Also, the slope intercept form of a line is y = mx + c,

Where, m is the slope of the line,

x + y = 2 ⇒ y = -x + 2

Thus, the slope of the line x + y = 2 is -1,

Let
`m_1` is the slope of the line that is perpendicular to x + y = 2,

By the above statement,

`m_1* -1=-1\implies m_1 = 1`

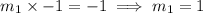
Suppose y = x + c is the line perpendicular to the line x + y = 2,

According to the question,

y = x + c is passes through the point (1,-6),

⇒ -6 = 1 + c ⇒ -6 - 1 = c ⇒ c = -7

Hence, the equation of the required line is,

y = x - 7

⇒ x - y = 7

Compare this with standard form of line ax+by=c

We get, a = 1, b = -1 and c = 7