Question

A certain sound level increases by an additional 20 db. By how much does the intensity increase?

Answer 1

The intensity increases by a factor 100.

Step-by-step explanation:

Given that,

Sound intensity = 20 db

We need to calculate the intensity factor

`F=10^{(LI)/(10)}`

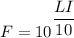
Where, LI = sound intensity

Put the value into the formula

`F=10^{(20)/(10)}`

`F=10^2`

`F=100`

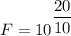
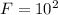
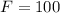
Hence, The intensity increases by a factor 100.