Question

Which statements accurately describe the function f(x) = 3 sqrt 18?

algebra II engenuity

Answer 1

The statements which accurately describe f(x) are

The domain is all real numbers ⇒ 1st answer

The initial value of 3 ⇒ 3rd answer

The simplified base is 3√2 ⇒ last answer

Explanation:

* Lets explain how to solve the problem

- The form of the exponential function is f(x) = a(b)^x, where a is the

initial value , b is the base and x is the exponent

- The values of a and b are constant

- The domain of the function is the values of x which make the function

defined

- The range of the function is the set of values of y that correspond

with the domain

* Lets solve the problem

∵
`f(x)=3(√(18)) ^(x)`

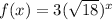
- The simplest form of is :

∵ √18 = √(9 × 2) = √9 × √2

∵ √9 = 3

∴ √18 = 3√2

∴
`f(x)=3(3√(2))^(x)`

∵
`f(x)=a(b)^(x)`

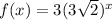
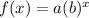
∴ a = 3 , b = 3√2

∴ The initial value is 3

∴ The simplified base is 3√2

- The exponent x can be any number

∴ The domain of the function is x = (-∞ , ∞) or {x : x ∈ R}

- There is no value of x makes y = 0 or negative number

∴ The range is y = (0 , ∞) or {y : y > 0}

* Lets find the statements which accurately describe f(x)

# The domain is all real numbers

∵ The domain is {x : x ∈ R}

∴ The domain is all real numbers

# The initial value is 3

∵ a = 3

∵ a is the initial value

∴ The initial value of 3

# The simplified base is 3√2

∵ b = √18

∵ b is the base

∵ The simplified of √18 is 3√2

∴ The simplified base is 3√2

- For more understand look to the attached graph