Answer:
Option B k > 0
Explanation:
we know that
Observing the graph
The slope of the line is positive
The y-intercept is negative
we have
![3y-2x=k(5x-4)+6\\ \\3y=5kx-4k+6+2x\\ \\3y=[5k+2]x+(6-4k)\\ \\y=(1)/(3)[5k+2]x+(2-(4)/(3)k)](https://img.qammunity.org/2020/formulas/mathematics/middle-school/ylphcciqgu0rbyxglpl9o88adalttknoxv.png)
The slope of the line is equal to
![m=(1)/(3)[5k+2]](https://img.qammunity.org/2020/formulas/mathematics/middle-school/n25cgr4nk1c2arc76pc8lhw6m68tnq0uxn.png)
Remember that the slope must be positive
so
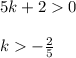
The value of k is greater than -2/5
Analyze the y-intercept
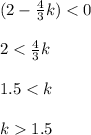
1.5 is greater than zero
so
the solution for k is the interval ------> (1.5,∞)
therefore
must be true
k > 0