Answer: 3
Explanation:
Given : The average time required to complete an accounting test :

Interval = (45, 60) minutes
In hour : Interval = (0.75, 1)
The cumulative distribution function for exponential function is given by :-
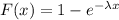
For
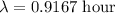
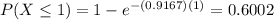
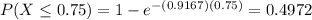
Then ,
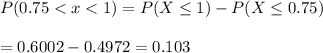
Now, the number of students from a class of 30 should be able to complete the test in between 45 and 60 minutes =
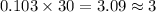
Hence, the number of students should be able to complete the test in between 45 and 60 minutes =3