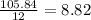Answer:
8.82 years.
Explanation:
Since, the monthly payment formula is,
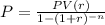
Where, PV is the present value of the loan,
r is the rate per month,
n is number of months,
Here,
PV = $ 25,000,
Annual rate = 5.2 % = 0.052 ⇒ Monthly rate, r =
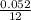
( 1 year = 12 months )
P = $ 295,
By substituting the values,
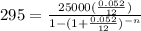
By the graphing calculator,
We get,
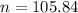
Hence, the time ( in years ) =