Answer:
Yes , S is a basis for
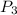 .
.
Explanation:
Given
S=
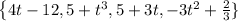 .
.
We can make a matrix
Let A=
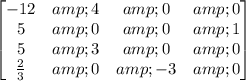
All rows and columns are linearly indepedent and S span
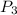 .Hence, S is a basis of
.Hence, S is a basis of
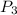
Linearly independent means any row or any column should not combination of any rows or columns.
Because a subset of V with n elements is a basis if and only if it is linearly independent.
Basis:- If B is a subset of a vector space V over a field F .B is basis of V if satisfied the following conditions:
1.The elements of B are linearly independent.
2.Every element of vector V spanned by the elements of B.