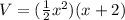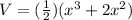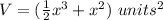Question

The oblique prism below has an isosceles right triangle base. what expression represents the volume of the prism in cubic units?

The answer is (A). Your welcome ​

Answer 1

ANSWER IS A

Explanation:

Answer 2

`V=((1)/(2)x^(3)+x^(2))\ units^(2)`

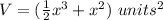
Explanation:

we know that

The volume of the oblique prism is equal to

`V=BH`

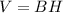
where

B is the area of the base

H is the height of the prism

Find the area of the triangular base

The area B is equal to

`B=(1)/(2)x^(2)\ units^(2)`

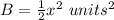
`H=(x+2)\ units` ---> the height must be perpendicular to the base

substitute

`V=((1)/(2)x^(2))(x+2)`

`V=((1)/(2))(x^(3)+2x^(2))`

`V=((1)/(2)x^(3)+x^(2))\ units^(2)`