Answer:
All the polar coordinates of point P are
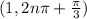 and
and
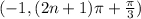 , where n is an integer.
, where n is an integer.
Explanation:
The given point is
 .... (1)
.... (1)
If a point is defined as
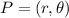 .... (2)
.... (2)
then the polar coordinates of point P is defined as

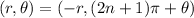
where, n is an integer and θ is in radian.
From (1) and (2) we get
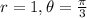
So, the polar coordinates of point P are
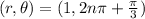
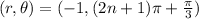
Therefore all the polar coordinates of point P are
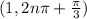 and
and
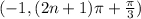 , where n is an integer.
, where n is an integer.