Question

Light of wavelength 608.0 nm is incident on a narrow slit. The diffraction pattern is viewed on a screen 88.5 cm from the slit. The distance on the screen between the fifth order minimum and the central maximum is 1.61 cm. What is the width of the slit?

Answer 1

The width of the slit is 0.167 mm

Step-by-step explanation:

Wavelength of light,
`\lambda=608\ nm=608* 10^(-9)\ m`

Distance from screen to slit, D = 88.5 cm = 0.885 m

The distance on the screen between the fifth order minimum and the central maximum is 1.61 cm, y = 1.61 cm = 0.0161 m

We need to find the width of the slit. The formula for the distance on the screen between the fifth order minimum and the central maximum is :

`y=(mD\lambda)/(a)`

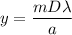
where

a = width of the slit

`a=(mD\lambda)/(y)`

`a=(5* 0.885\ m* 608* 10^(-9)\ m)/(0.0161\ m)`

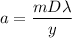
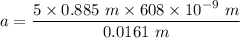
a = 0.000167 m

`a=1.67* 10^(-4)\ m`

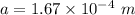
a = 0.167 mm

So, the width of the slit is 0.167 mm. Hence, this is the required solution.