Question

The acceleration of a particle is given by a = 6t - 28, where a is in meters per second squared and t is in seconds. Determine the velocity and displacement as functions of time. The initial displacement at t = 0 is s0= -8 m, and the initial velocity is v0 = 6 m/s. After you have the general expressions, evaluate these expressions at the indicated times.

Answers:

At t = 5.8 s, s = m, v = m/s
At t = 12.7 s, s = m, v = m/s

Answer 1

Velocity, V = 3t²- 28t+6

Displacement, s = t³ - 14t²+6t -8

At t = 5.8 s

s = -249.05 m

v = -55.48 m/s

At t = 12.7 s

s = -141.48 m

v = 134.27 m/s

Step-by-step explanation:

We have acceleration of a particle is given by a = 6t - 28

Velocity

`v=\int adt=\int (6t - 28)dt=3t^2-28t+C`

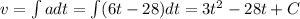
At t = 0 we have v₀ = 6 m/s

v₀ = 6 = 3 x 0 ²-28 x 0+C

C = 6

So velocity, V = 3t² - 28t+6

Displacement

`s=\int vdt=\int (3t^2-28t+6)dt=t^3-14t^2+6t+C`

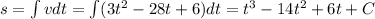
At t = 0 we have s₀ = -8 m

s₀ = -8 = 0³ + 14 x 0² + 6 x 0 + C

C = -8

So displacement, s = t³ - 14t²+6t -8

At t = 5.8 s

s = 5.8³ - 14 x 5.8²+6 x 5.8 - 8 = -249.05 m

v = 3 x 5.8² - 28 x 5.8 + 6 = -55.48 m/s

At t = 12.7 s

s = 12.7³ - 14 x 12.7²+6 x 12.7 - 8 = -141.48 m

v = 3 x 12.7² - 28 x 12.7 + 6 = 134.27 m/s