Answer with explanation:
Size of the sample = n =225
Mean[\text] \mu[/text]=48.5
Standard deviation [\text] \sigma[/text]= 1.8
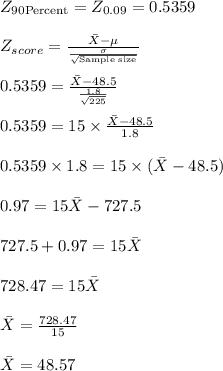
→Given Confidence Interval of Mean =48.8
→Calculated Mean of Sample =48.57 < 48.8
So, the value of Sample mean lies within the confidence interval.